Un cours sur le produit scalaire dans l'espace, avec au programme : définition, propriétés, orthogonalité, équations cartésiennes et distances dans l'espace.
1 - Définitions du produit scalaire dans l'espace
Nous attaquons maintenant la partie dans l'espace. Vous allez voir, c'est presque pareil, à un axe prés.
Définition
Produit scalaire dans l'espace
Soient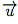 et
et 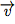 deux vecteurs de l'espace.
deux vecteurs de l'espace.
Le produit scalaire de
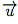 et
et 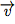 dans l'espace n'est rien d'autre que le produit scalaire de
dans l'espace n'est rien d'autre que le produit scalaire de 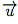 et
et 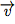 dans le plan contenant ces deux vecteurs.
dans le plan contenant ces deux vecteurs.
Prenons un point A de l'espace. Il existe donc deux points, B et C, tels que : 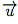 =
=  et
et 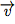 =
=  .
.
Il existe forcément un plans qui contient les points A, B et C puisqu'un plan est formé par trois points distincts.
Le produit scalaire de 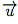 et
et 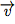 dans l'espace est donc le produit scalaire de
dans l'espace est donc le produit scalaire de 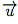 et
et 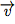 dans le plan contenant ces deux vecteurs.
dans le plan contenant ces deux vecteurs.
Pour les coordonnées des vecteurs, c'est pareil que dans le plan, à ça prés.
Définition
Coordonnées et produit scalaire dans l'espace
Soient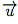 (x; y; z) et
(x; y; z) et 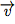 (x'; y'; z') deux vecteurs de l'espace.
On a alors :
(x'; y'; z') deux vecteurs de l'espace.
On a alors : 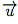 .
.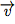 = xx' + yy' + zz'
= xx' + yy' + zz'
Exemple
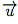 (3; -1; 0) et
(3; -1; 0) et 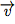 (-2; 1; 5) dans l'espace.
(-2; 1; 5) dans l'espace.
Le produit scalaire de ces deux vecteurs est :
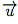 .
.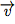 = 3 × (-2) + (-1) × 1 + 0 × 5 = -6 - 1 + 0 = -7
= 3 × (-2) + (-1) × 1 + 0 × 5 = -6 - 1 + 0 = -7
Et si ce produit scalaire est nul, on a aussi des vecteurs orthogonaux dans l'espace ?
Alors, oui. J'y reviens juste après ça. Ne bougez pas.
2 - Propriétés du produit scalaire dans l'espace
Les propriétés du produit scalaires dans l'espace sont les mêmes que celles dans le plan.
3 - Orthogonalité et produit scalaire dans l'espace
Nous allons maintenant parler d'orthogonalité dans l'espace.
Propriété
Orthogonalité et produit scalaire dans l'espace
Soient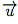 et
et 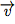 deux vecteurs de l'espace.
deux vecteurs de l'espace.
Les vecteurs
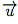 et
et 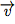 sont orthogonaux si
sont orthogonaux si 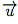 .
.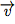 = 0.
= 0.
Soit un vecteur
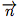 de l'espace et un point A.
de l'espace et un point A.
L'ensemble des points M de l'espace vérifiant
 .
.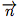 = 0 est un plan passant par A et de vecteurs normal
= 0 est un plan passant par A et de vecteurs normal 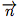 .
.
Deux plans
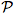 et
et 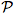 ' de vecteurs normaux respectifs
' de vecteurs normaux respectifs 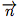 et
et 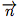 ' sont perpendiculaires si
' sont perpendiculaires si 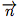 .
.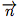 ' = 0.
' = 0.
Si on a une droite, de vecteur directeur 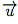 et un plan
et un plan 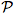 , alors le vecteur
, alors le vecteur 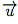 est normal à ce plan s'il est perpendiculaire à toutes les droites contenues dans le plan.
est normal à ce plan s'il est perpendiculaire à toutes les droites contenues dans le plan.
Exemple
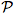 et
et 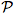 ' de vecteurs normaux respectifs
' de vecteurs normaux respectifs 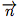 (-5;0;2) et
(-5;0;2) et 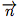 '(-2; 6; -5) sont perpendiculaires.
'(-2; 6; -5) sont perpendiculaires.
En effet, calculons le produit scalaire de
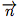 et
et 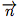 '.
'.
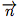 .
.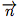 ' = (-5) × (-2) + 0 × 6 + 2 × (-5) = 10 + 0 - 10 = 0
' = (-5) × (-2) + 0 × 6 + 2 × (-5) = 10 + 0 - 10 = 0Donc, les vecteurs
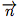 et
et 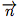 ' sont orthogonaux, et donc les plan
' sont orthogonaux, et donc les plan 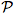 et
et 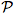 ' sont perpendiculaires.
' sont perpendiculaires.
Exemple
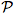 passant par A(2; 3; 1) et de vecteur normal
passant par A(2; 3; 1) et de vecteur normal 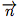 (-2; 1; 3).
(-2; 1; 3).
Pour tout point M(x; y; z) de l'espace,
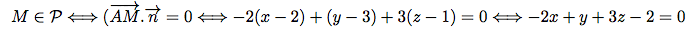
Donc, l'équation cartésienne du plan
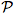 passant par A(2; 3; 1) et de vecteur normal
passant par A(2; 3; 1) et de vecteur normal 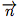 (-2; 1; 3) est : -2x + y + 3z - 2 = 0.
(-2; 1; 3) est : -2x + y + 3z - 2 = 0.
4 - Applications du produit scalaire dans l'espace
a - Equation cartésienne dans l'espace
On va définir l'équation cartésienne d'un plan.
Définition
Equation cartésienne dans l'espace
Tout plan de l'espace, de vecteur normal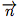 (a; b; c) admet une équation cartésienne de la forme ax + by + cz + d = 0.
(a; b; c) admet une équation cartésienne de la forme ax + by + cz + d = 0.
Réciproquement, toute équations de la forme ax + by + cz + d = 0 est l'équation d'un plan de l'espace de vecteur normal
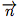 (a; b; c).
(a; b; c).
Exemple
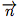 (3; 0; -4).
(3; 0; -4).
b - Distance d'un point à un plan
On va parler maintenant de distance d'un point à un plan.
Propriété
Distance d'un point à un plan
Soient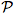 le plan d'équation ax + by + cz + d = 0 et A(xA; yA; zA) un point de l'espace.
le plan d'équation ax + by + cz + d = 0 et A(xA; yA; zA) un point de l'espace.
La distance du point A au plan
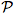 est la distance AH, avec H le projeté orthogonal de A sur
est la distance AH, avec H le projeté orthogonal de A sur 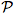 .
.
On a :
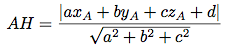
C'est la même formule que dans le plan, en rajoutant un axe, l'axe z.
Exemple
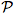 le pan d'équation -x + 2y + 5z + 2 = 0 et A(5; 2; -1) un point de l'espace.
le pan d'équation -x + 2y + 5z + 2 = 0 et A(5; 2; -1) un point de l'espace.
La distance de A à
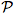 est donc la distance AH, avec H le projeté orthogonal de A sur
est donc la distance AH, avec H le projeté orthogonal de A sur 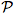 .
.

Quelques exercices sur Produit scalaire dans l'espace :
- Exercice : Equation cartésienne d'un plan
- Exercice : Distance d'un point à un plan
- Exercice : Equation cartésienne d'une sphère
- Exercice : Orthocentre d'un triangle
- Exercice : Equation cartésienne de plans
- Exercice : Equation cartésienne de sphères
- Exercice : Calcul de longueur, équation cartésienne, aire et volume